when i started doing illustration for calculus BLUE, i had insufficient appreciation for the technicalities of hidden lines. it’s taken me a long time to really understand how they can best be used, and i’m still learning. here are some simple examples from the exercises in the e-text version of volume 4, used for induced orientations in stokes’ theorem.
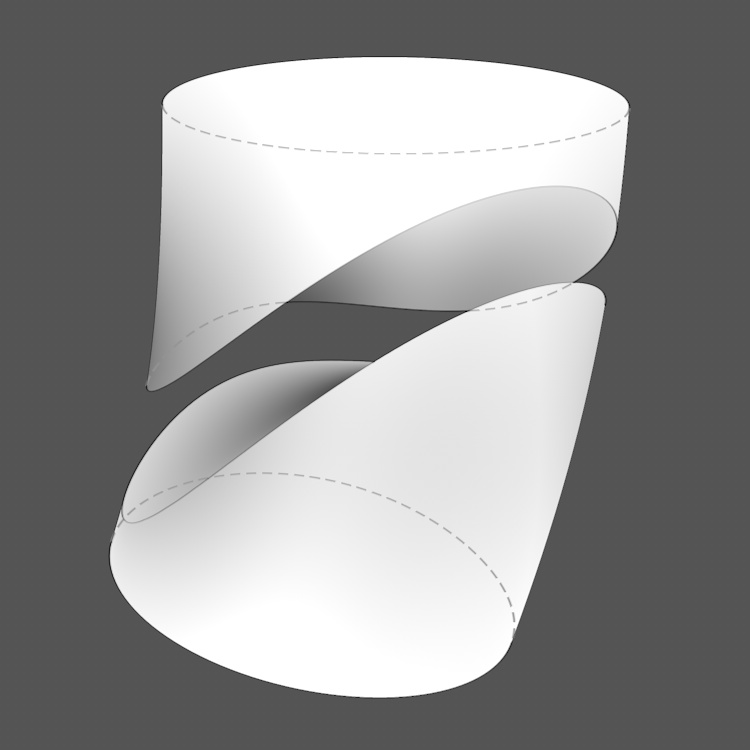


none of these are particularly good or deep examples. yet the combination of the hidden lines and the shading makes such a difference in grasping the surface. when we get to colors, thicknesses, and intersections, there will be much more to discuss…